

Refraction Class – X Part – 2
tag:
Share:
Admin Koushi


Deviation of a ray during refraction: Whenever a ray of light travels from one medium to another medium it deviates from its path. The angle between the refracted ray and the direction of the incident ray gives the measurement of deviation.
Let us consider AO is the incident ray and OB is the refracted ray where i and r are the angle of incidence and angle of refraction respectively.
When light travels from rarer medium to denser medium, ![]() BOC is the angle of deviation. So,
BOC is the angle of deviation. So, ![]() BOC =
BOC = ![]() CON/ –
CON/ – ![]() BON/
BON/
Or, ![]() =
= ![]() AON –
AON – ![]() BON/
BON/
Or, ![]() = i – r
= i – r

When light travels from denser medium to rarer medium, ![]() BOC is the angle of deviation. So,
BOC is the angle of deviation. So, ![]() BOC =
BOC = ![]() BON/ –
BON/ – ![]() CON/
CON/
Or, ![]() =
= ![]() BON/ –
BON/ – ![]() AON
AON
Or, ![]() = r – i
= r – i

Example: A ray of light is incident on a plane of a glass slab from air at an angle 450 and due to refraction the ray is deviated through an angle 150. Calculate the refractive index of the glass slab.
As light travels from rarer medium to denser medium, then angle of deviation is ![]() = 450 – 150 = 300
= 450 – 150 = 300
Using Snell’s law we get, refractive index of glass slab ![]() =
= ![]() =
= ![]() = 1.414.
= 1.414.
Principle of reversibility of light: When a light travels from medium 1 to medium 2 along the path AOB, AO is the incident ray and OB is the refracted ray. Using Snell’s law ![]() = 1µ2 —–(1)
= 1µ2 —–(1)
Where 1µ2 is the refractive index of a medium 2 with respect to medium 1.
When the path of ray of light is reversed, BO is the incident ray and OA is the refracted ray. Using Snell’s law ![]() = 2µ1 —–(2)
= 2µ1 —–(2)
Where 2µ1 is the refractive index of a medium 1 with respect to medium 2.
From equation (1) and equation (2) we get, ![]()
![]()
![]() = 1µ2
= 1µ2 ![]() 2µ1
2µ1
Or, 1µ2 ![]() 2µ1 = 1
2µ1 = 1
![]() 1µ2 =
1µ2 = ![]() .
.

Refraction through a glass slab: Let us consider a rectangular glass slab of refractive index µ and thickness t placed in air. A ray of light AB incident at B with angle of incidence i. After refraction, the ray of light bends towards the normal and goes along BC such that r is the angle of refraction.
Using Snell’s law at point B we get, ![]() = aµg —–(1)
= aµg —–(1)
At point C, the ray of light again suffers refraction and emerges out of the glass into air along CD. For refraction at C, r is the angle of incidence and i/ is the angle of refraction. Using Snell’s law at point C we get, ![]() = gµa ——(2)
= gµa ——(2)
From equation (1) and equation (2) we get, = ![]()
![]()
![]() = aµg
= aµg ![]() gµa
gµa
Or, ![]() = 1 [as aµg =
= 1 [as aµg = ![]() ]
]
Or, sin i = sin i/
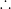 i = i/
i = i/
So, the ray of light incident on a parallel glass slab emerge out parallel to the incident ray.
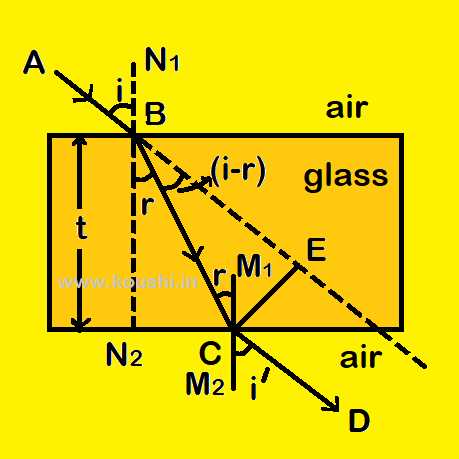
Refraction through compound slabs: Let us consider two parallel slabs (slab A and slab B) of absolute refractive index µA and µB respectively are placed one over another as shown in figure. C is the medium above and below the slabs with absolute refractive index µC. Let PQ is the incident ray on slab A from medium C and ST is the emergent ray from slab B in medium C. As the slabs are parallel and incident and emergent medium are same then the angle of incidence and angle of emergence are same (say i).
Using Snell’s law at point Q when ray PQ passes from medium C to medium A we get, ![]() = CµA ——-(i)
= CµA ——-(i)
Using Snell’s law at point R when ray QR passes from medium A to medium B we get, ![]() = AµB ——-(ii)
= AµB ——-(ii)
Using Snell’s law at point S when ray RS passes from medium B to medium C we get, ![]() = BµC ——-(iii)
= BµC ——-(iii)
Multiplying equations (i), (ii) and (iii) we get,
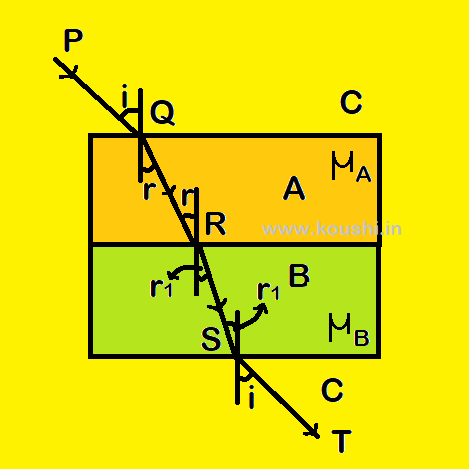
![]()
![]()
![]()
![]()
![]() = (CµA)
= (CµA) ![]() ( AµB)
( AµB) ![]() ( BµC)
( BµC)
Or, (CµA) ![]() ( AµB)
( AµB) ![]() ( BµC) = 1
( BµC) = 1
Or, AµB = ![]() =
= ![]() ——(iv)
——(iv)
If medium C is air then CµA = µA and CµB = µB then from equation (iv) we get, AµB = ![]() .
.
Example: Refractive index of water with respect to air is 1.33, refractive index of oil with respect to water is 1.35 and that of glass with respect to oil is 0.65. What is the refractive index of glass with respect to air?
Refractive index of water with respect to air is ![]() = 1.33
= 1.33
Refractive index of oil with respect to water is ![]() = 1.35
= 1.35
Refractive index of glass with respect to oil is ![]() = 0.65
= 0.65
We know that ![]()
![]()
![]()
![]()
![]()
![]()
![]() = 1
= 1
Or, ![]()
![]()
![]()
![]()
![]() =
= 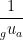 =
= ![]()
![]()
![]() = 1.33
= 1.33![]() 1.35
1.35![]() 0.65 = 1.167.
0.65 = 1.167.
Click the button to go to the next part of this chapter.
Click the button to go to the previous part of this chapter.
© 2018 – 2025 Koushi All Rights Reserved